

The two scatter plots below show that there is a strong correlation between TID and both Ave. Price and Ave. Adver.
TID variables
 |
 |
 |
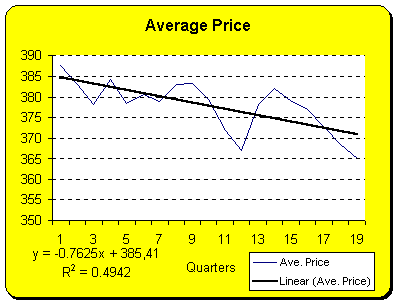
The three line graphs show that there are
trends in the variables over time. The upward trend in TID and the downward trend in Ave.
Price are much stronger then the upward trend in Ave. Adv. Only about 27% of the data can
be explained by the linear trendline. The two scatter plots below show that there is a strong correlation between TID and both Ave. Price and Ave. Adver. |
 |
 |
The correlation matrix gives important data on how the variables are related to one another:
| Quarter | Avg_Price | Avg_Adv | TID | |
| Quarter | 1.000 | |||
| Avg_Price | -0.703 | 1.000 | ||
| Avg_Adv | 0.522 | -0.749 | 1.000 | |
| TID | 0.695 | -0.889 | 0.882 | 1.000 |
The correlation matrix shows that TID is correlated with all of the variables. Unfortunately, some of the independent variables are highly correlated to each other, casting a doubt on their being independent.
A regression analysis of the three variables paints a telling picture. See the table below.
| Regression Analysis for TID using Quarter, Average Price and Average Advertising as independent variables | ||||||||
| Regression Statistics | ||||||||
| Multiple R | 0.9523 | |||||||
| R Square | 0.9069 | Taking a quick look at the table, it is obvious, that including Quarter in the analysis will probably result in a Type I error, since the P-value for Quarter is almost 22%. It is best not to include it. | ||||||
| Adj. R Squ. | 0.8883 | |||||||
| Regression Coefficients | ||||||||
| Coefficients | Stand. Err. | t Stat | P-value | |||||
| Intercept | 130249.28 | 50620.07 | 2.57 | 0.021 | ||||
| Quarter | 123.23 | 102.99 | 1.28 | 0.219 | ||||
| Avg. Price | -358.61 | 122.18 | -2.94 | 0.010 | ||||
| Avg. Adv. | 0.26 | 0.06 | 4.16 | 0.001 | ||||
By excluding the Quarter variable, the data becomes valid for constructing an equation for TID. See table below.
| Regression Analysis for TID using Average Price and Average Advertising as independent variables | ||||||||
| Regression Statistics | ||||||||
| Multiple R | 0.9470 | |||||||
| R Square | 0.8967 | After excluding the Quarter variable, the other variables P-values increased. Now all P-values are at an acceptable level. | ||||||
| Adj. R Squ. | 0.8838 | |||||||
| Regression Coefficients | ||||||||
| Coefficients | Stand. Err. | t Stat | P-value | |||||
| Intercept | 164336.17 | 43962.47 | 3.74 | 0.002 | ||||
| Avg. Price | -445.16 | 103.94 | -4.28 | 0.001 | ||||
| Avg. Adv. | 0.26 | 0.06 | 4.07 | 0.001 | ||||
Based on the table above, the following equation can be created:
TID=164336.17-445.16*Avg. Price+0.26*Avg. Adv.
This equation explains roughly 90% of all TID values based on Avg. Price and Avg. Adv.